3. How high could a man jump on the Moon?

This one is completely trivial; I’m writing it just for a little intellectual fun.
Since the days of Apollo, everyone has known that the Moon has roughly one sixth of the Earth’s gravity, and therefore that an astronaut standing on it has one sixth of the weight which he has on Earth. More correctly, the acceleration due to gravity on the surface of the Moon – the rate at which a falling object accelerates - is one sixth of that on the surface of the Earth.
The mass of any object is always the same, whether it’s on Earth, on the Moon or in a spacecraft in orbit. But its weight depends on where it is; an object’s weight is the gravitational force acting on it – more correctly, the mutual gravitational force between it and the planet on which it’s standing – which is equal to its mass multiplied by the acceleration due to gravity. So an astronaut on the Moon has the same mass as he does on Earth, but one sixth of the weight.
In everyday language, we usually express weight in the same units as mass, such as pounds or kilograms – but in physics, this isn’t correct. As stated above, weight is in fact a force, so it’s measured in the units of force, newtons ( or foot-pounds in old-fashioned imperial units ). The acceleration due to gravity, at the surface of the Earth, is 9.8 metres per second squared ( 9.8 ms^-2 ), so a mass of one kilogram has a weight of 9.8 newtons ( 9.8 N ). It’s also valid to say it has a weight of “one kilogram force”.
Note that, while I’m specifically talking about planetary surfaces here, this does not mean that “there is no gravity in space”!!! I’ll address that ridiculous misconception in a later essay.
It’s often said that a man could jump six times as high on the Moon as he can on Earth. Whether that’s correct depends on how you define “jump”. If you just mean how high he could jump vertically from a standstill, then it is correct – but if you mean an athletics-style high jump, then it isn’t that simple.
The world high jump record is a tiny fraction over eight feet. In fact, at the time of writing, the world record has stood for 23 years, so it must have just about reached the maximum which is physically possible! So a champion athlete on the Moon ( in the pressurised environment of a future lunar base, naturally! ) would be able to clear a height of about six times eight, or 48 feet, right? Wrong!
In physics, in any calculation involving motion and forces, any object can be considered as a point mass located at its centre of gravity – more correctly called its centre of mass. This is usually not the same as its geometric centre, as it depends on how the object’s mass is distributed. The centre of gravity of a human being is slightly higher than half of his or her height – around the height of the navel - as there is more mass in the upper half of the body than in the lower half. For a man of, say, six feet four inches ( well, champion high jumpers are likely to be of above average height! ), standing upright, his centre of gravity is around three and a half feet above the ground. I’m deliberately using imperial units here, as most British and American readers will find it easier to visualise a person’s height in feet than in metres!
A high jumper, of course, uses the “Fosbury Flop” technique, as in Fig. 3. At the highest point of his trajectory, his body is in a horizontal position, so his centre of gravity is only a few inches above the bar.

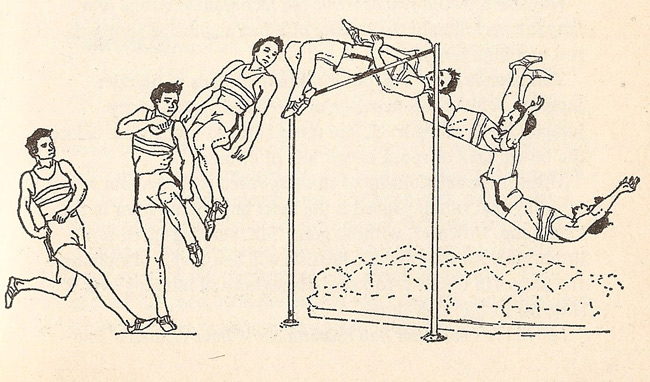
Fig. 3

So picture our athlete clearing a bar at a height of eight feet. When he takes off from an upright position, his centre of gravity is 3.5 feet above the ground, and as he clears the bar, it’s about 8.5 feet above the ground. So while he has cleared the bar at eight feet, he has only raised his centre of gravity through a height of five feet.
Now picture him doing the same on the Moon. Under lunar gravity, he would be able to raise his centre of gravity by six times five, or thirty feet. Add that initial 3.5 feet – the height of his centre of gravity while standing – and we see that the lunar high jump record would be a “mere” 33.5 feet, rather than 48! That would still be pretty impressive!

Previous page Next page

Return to Contents